Last month I introduced the value of non-linear regression analysis in your appraisal practice. These relatively simple tools allow an analyst to describe how the market behaves, over multiple orders of magnitude, using math that is no more complex than a high-school algebra class. Typical linear analysis techniques are only valid over small changes in unit size (acres, s/f, loading docks...) commonly described as 10% larger or smaller than the subject. If all your comparable data fall within this range, then you operate in a market that is much more active than mine.
Expanding your practice to include non-linear techniques, especially those involving exponential decay, allows you to describe the behavior of buyers and sellers over a much wider range in variation. As an example I will re-introduce the data set from last month; a land market in rural Vermont, sorted only by including those arms' length sales with a single-unit residential highest and best use, no other discriminating factor was applied to sort the data in any way.
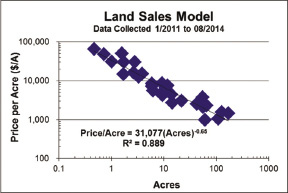
The resulting best-fit model is an exponential decay; Price/Acre = 31,077(acres)-0.65; which explains 89% of all the variation in price/acre over changes in parcel size that cover four orders of magnitude. The best-fit line above appears straight only because the axes above are in log-log format where each change in order of magnitude is represented as a fixed distance on the graph. If these terms are bringing back fond memories of high school math I would encourage you to use an internet search where you will find copious information on both exponential decay and log-log graphing.
In my practice any operating market that may be expressed as a price/unit ($/acre, $/parking space) is likely to follow a pricing model that is best expressed with an exponential decay. This is not as complicated as it sounds; an exponential decay is simply that where the change in price/unit gets smaller and smaller as the unit count gets bigger and bigger. I like a grocery analogy; the price you pay for difference between one and two bananas is much larger than the price you pay for the difference between the 10,000 and the 10,001st banana. Almost all real estate markets behave this way when expressed as price/unit, and those that don't are a key to the analysis that the market is behaving on a price/property basis; commonly associated with an extremely desirable amenity such as ski on /ski off location or dramatic mountain view.
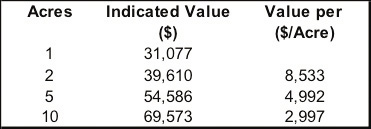
The beauty in resolving a market to an exponential decay model is that adjustment for differences in unit count are immediately apparent. Using the data set above, the best fit line tells us that the first acre sells for $31,077, the second for $8,533, the fifth for $4,992, and the 10th for $2,997. Changes in price/unit fall as the unit count increases; which is simple, expected based on efficiencies of scale, and explains the economic incentive in subdivision.
Remember the 80/20 rule the old appraiser in the office tried to drum into you when you started; 80% of the value is in the first 20% of the site; he was simply expressing an exponential decay in the behavior of the market. This also explains why a property that sells for $200 per s/f only requires per s/f adjustments of $40 per s/f to properly explain the behavior of buyers and sellers. You were practicing non-linear regression and exponential decay models all along and did not know it!
These techniques appear complicated and under USPAP you are required to understand and properly employ them. But once you are familiar with the math that underlies their application and with modern spreadsheet software the entire process of gathering data, modeling a best fit line and applying a non-linear adjustment to the comparables may be automated. Non-linear analysis is simply a quantification of methods you used since starting your practice; the mathematics involve only high-school algebra and belong in your appraisal toolbox.
Sean Sargeant, MAI, SRA is the president of Vermont chapter of the Appraisal Institute, Rutland, VT.